Subtracting polynomials

On this post we explain how to do the subtraction of polynomials. Also, you will find several examples and step-by-step solved exercises of subtractions of polynomials.

How to subtract polynomials

To subtract two polynomials, subtract the terms of the polynomials that are like terms. That is, the subtraction of polynomials consists of subtracting the terms that have the same variables and the same exponents.

In mathematics, you can calculate the subtraction of polynomials using two different methods: the horizontal method or the vertical method. Below you have the explanation of both procedures.

Horizontal subtraction of polynomials

Next we are going to see how to subtract two polynomials horizontally by solving an example:

• Calculate the following subtraction of two polynomials:

The monomials of the first parentheses remain the same, but we have to change the sign on the terms of the second parentheses since they have a minus sign in front:

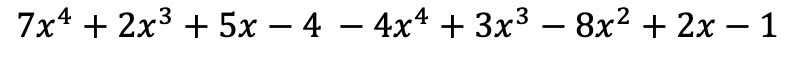
And now we combine the terms that have the same variables (letters) with the same exponents (like terms). Terms that are not like terms cannot be added or subtracted.

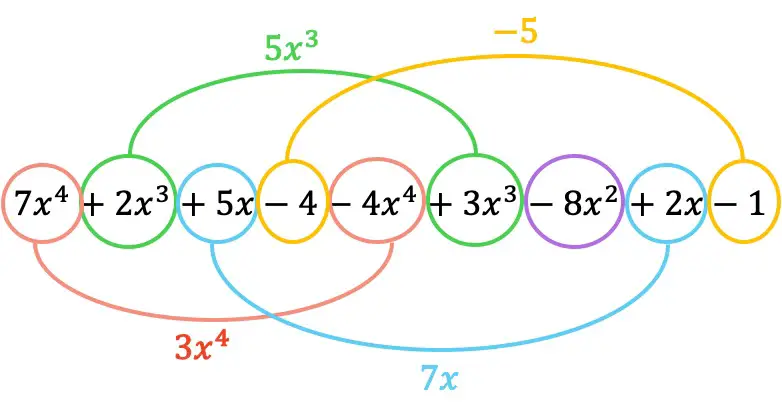
So the polynomial resulting from the subtraction is:

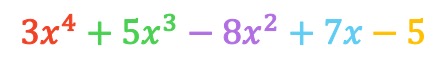
Vertical subtraction of polynomials

We have just seen how polynomials are subtracted horizontally, but now we are going to see the other method that exists to do subtraction of polynomials: subtracting polynomials vertically.

So, let’s see what this method of subtracting polynomials consists of using an example. And so that you can see the differences between the two methods, we will subtract the same polynomials as in the previous example:

• Perform the subtraction of the following two polynomials:

The first thing we must do to find any vertical subtraction of polynomials is to put one polynomial below the other, so that the like terms of the two polynomials are aligned by columns:

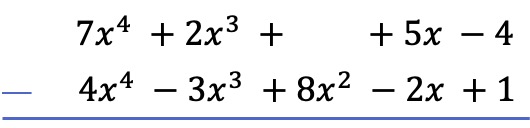
Note: if a polynomial does not have a term of a certain degree, we must leave the space blank. For example, the polynomial has no second degree monomial, so there is an empty space in its place.

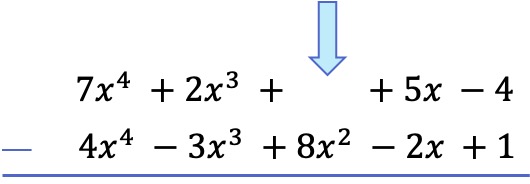
Although polynomials could now be subtracted directly, it is quite easy to go wrong with the signs if we do it this way. Therefore, to perform the subtraction of polynomials it is better to change the sign of all the terms of the subtrahend polynomial and then do the addition. Since subtracting a polynomial is equivalent to adding its opposite polynomial.

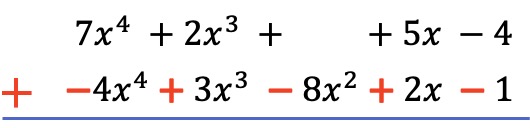
And once we have arranged all the terms in order from highest to lowest degree and have negated the terms of the polynomial below, we add the coefficients of each column keeping the variables and exponents the same:

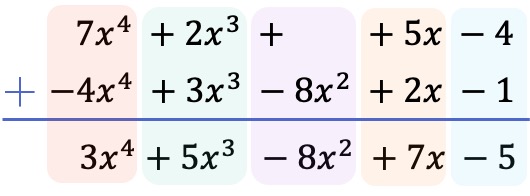
Therefore, the result obtained from the subtraction of the 2 polynomials is:

As you can see, we have obtained the same result with both methods, so you can use the one that you prefer.

In case the last step has not been clear to you, I leave you with the explanation of how to do the addition of polynomials, in fact, it is very important that you master the addition of polynomials to be able to subtract polynomials well. On the linked post you will also find examples and practice problems on adding polynomials and, furthermore, you will see the differences between the addition and the subtraction of polynomials.

Practice problems on subtracting polynomials

So that you can practice, below you have exercises with solutions of subtraction of polynomials. If you have any questions about any exercise, you can ask them in the comments section.

Problem 1

Subtract the following polynomials:

In this case, we will subtract the two polynomials vertically. To do this, we first order the polynomials by degree, then we change the sign of the terms of the subtrahend polynomial and, finally, we add the monomials that are in the same column:

 

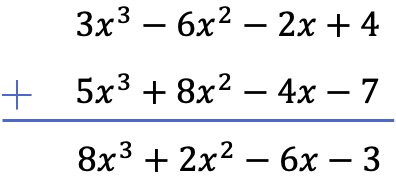
Problem 2

Solve the following subtraction of polynomials:

Subtracting two polynomials is the same as adding the opposite of the subtrahend polynomial to the minuend polynomial. Thus, we change the sign of the subtrahend polynomial and add the polynomials:

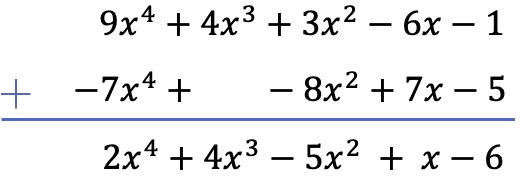
Note that in this particular case an empty space must be left in the column of degree 3 of the second polynomial, since it does not have a third degree term.

 

Problem 3

Find the following subtraction of polynomials:

In this case, we will solve the subtraction of the two polynomials vertically. So first we put the polynomials in order from highest to lowest degree, then we change the sign of the terms of the subtrahend polynomial, and finally we add the like terms:

 

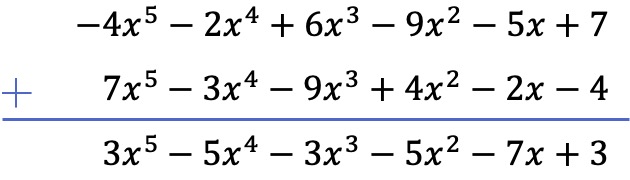
Problem 4

Simplify the following operation with polynomials:

In this case, 3 polynomials are involved in the operation, of which 2 are subtracting. So to solve the operation we will change the sign of all the terms of the two polynomials that are subtracted and then we will add the polynomials.